光栅化(Rasterization Cont)

关于

Antialiasing and Z-Buffering (反走样(抗锯齿)和深度缓冲)

锯齿的学名:走样

• Antialiasing (反走样)
  • Sampling theory (采样理论)
  • Antialiasing in practice (实践中的反走样 )
• Visibility / occlusion (可见性/遮挡)
  • Z-buffering

Sampling Artifacts (Errors / Mistakes / Inaccuracies) in Computer Graphics (采样时会出现的问题)

Artifacts due to sampling - “Aliasing” (采样产生的伪影——“混叠”)

1. Jaggies (Staircase Pattern) 锯齿(楼梯形状)

2. Moiré Patterns in Imaging (摩尔纹)

Skip odd rows and columns (跳过奇数行和奇数列)

3. Wagon Wheel Illusion (False Motion) (车轮错觉(假动作))

人眼在时间上的采样跟不上运动的速度

4. [Many more]...

Behind the Aliasing Artifacts (原因)

• Signals are changing too fast (high frequency), but sampled too slowly (信号变化太快(高频)但采样太慢)

Antialiasing (抗锯齿)

Antialiasing Idea: Blurring (Pre-Filtering) Before Sampling (抗锯齿方法: 采样之前做模糊(预滤波))

Point Sampling vs Antialiasing (点采样 vs 抗锯齿)

---

---

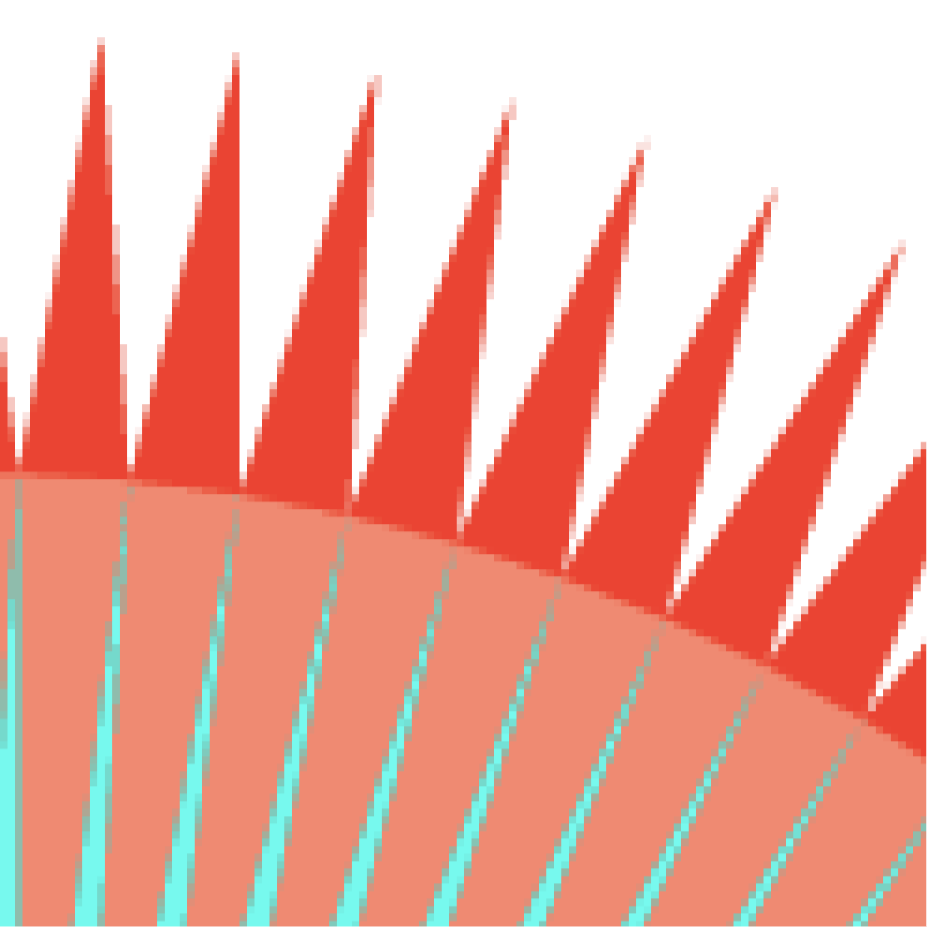
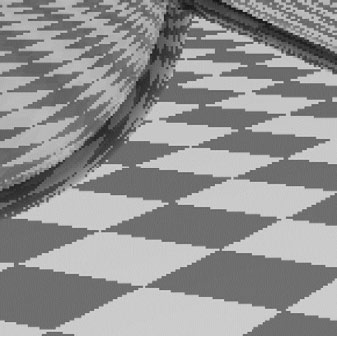
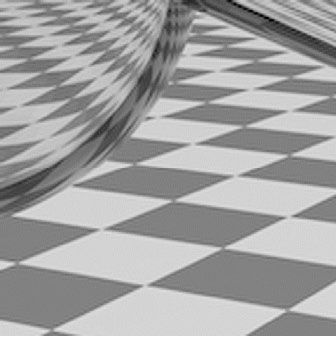
Antialiasing vs Blurred Aliasing

Sample then filter, WRONG!

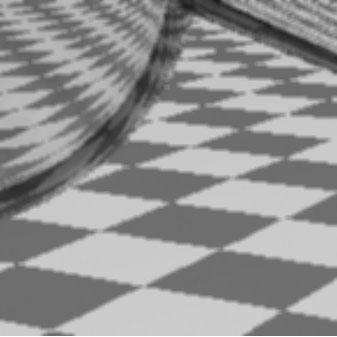
But Why?

1. Why undersampling introduces aliasing?
2. Why pre-filtering then sampling can do antialiasing?

Let’s dig into fundamental reasons And look at how to implement antialiased rasterization

Frequency Domain (频域)

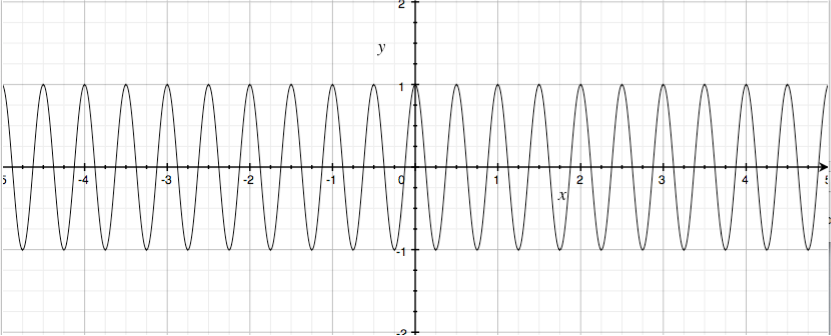
Sines and Cosines

$$\cos 2\pi x$$$$\sin 2\pi x$$

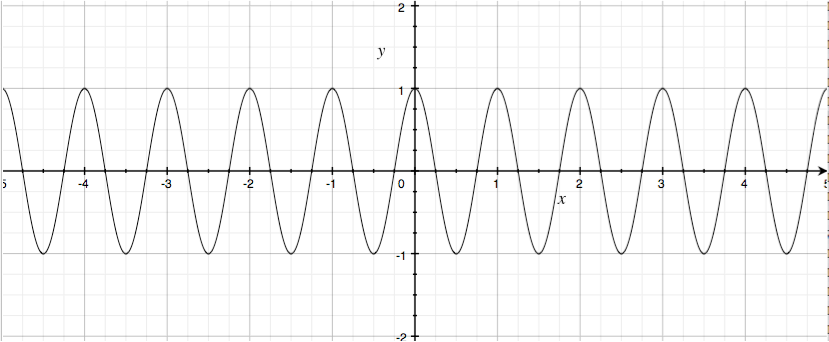
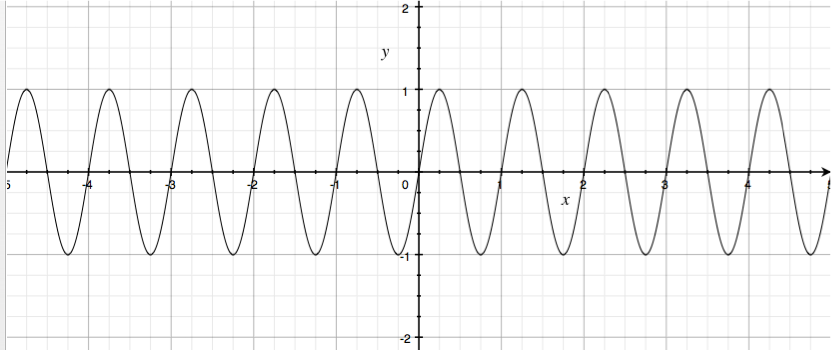
Frequencies (频率)

$$\cos 2\pi x$$$$f=1$$$$\cos 4\pi x$$$$f=2$$

---

$$\cos 2\pi fx$$$$f=\frac{1}{T}$$

Fourier Transform (傅里叶变换)

Fourier Transform (傅里叶变换)

• Represent a function as a weighted sum of sines and cosines (将函数表示为正弦和余弦的加权和)

$$f(x)=\frac{A}{2}+\frac{2A\cos (t\omega )}{\pi }-\frac{2A\cos (3t\omega )}{3\pi }+\frac{2A\cos (5t\omega )}{5\pi }-\frac{2A\cos (7t\omega )}{7\pi }+...$$

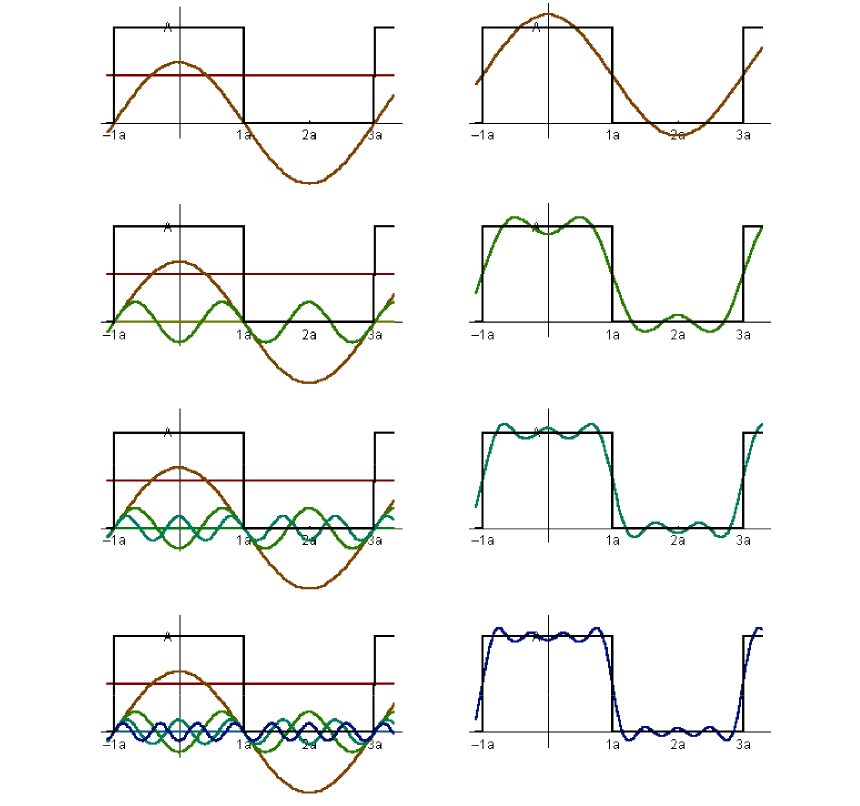
• Fourier Transform Decomposes A Signal Into Frequencies (傅里叶变换会将时域转化为频域)

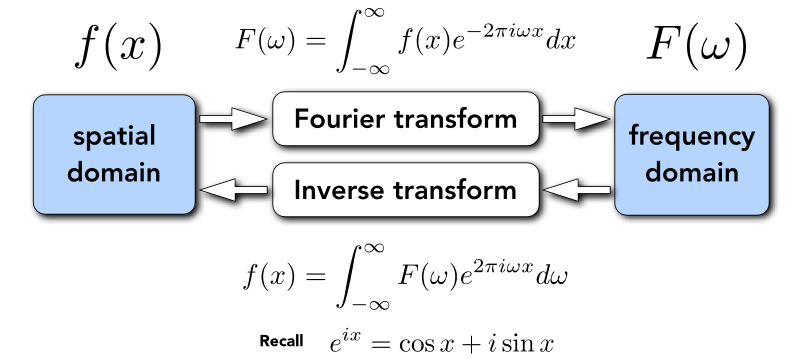
• Higher Frequencies Need Faster Sampling (更高的频率需要更快的采样)

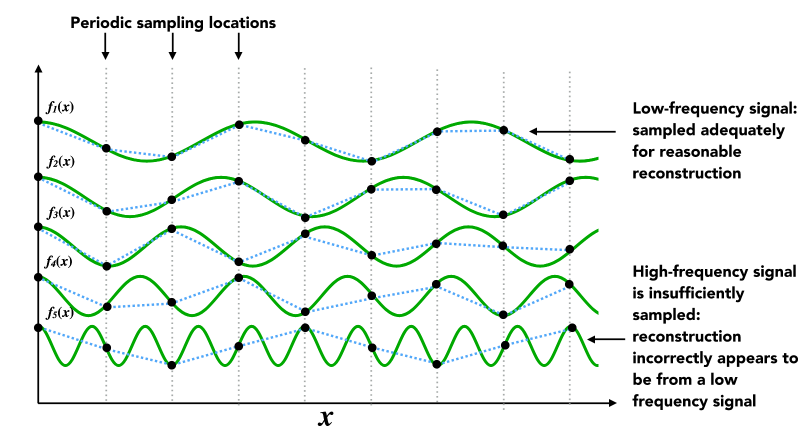
• Undersampling Creates Frequency Aliases
  • High-frequency signal is insufficiently sampled: samples erroneously appear to be from a low-frequency signal (高频信号采样不足:采样错误地显示为来自低频信号)
  • Two frequencies that are indistinguishable at a given sampling rate are called “aliases” (在给定采样率下无法区分的两个频率称为“别名”)

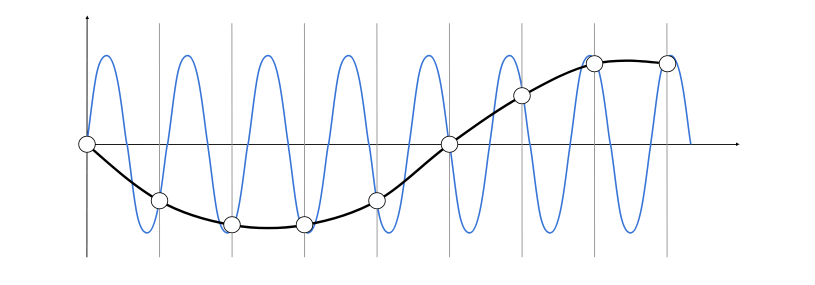
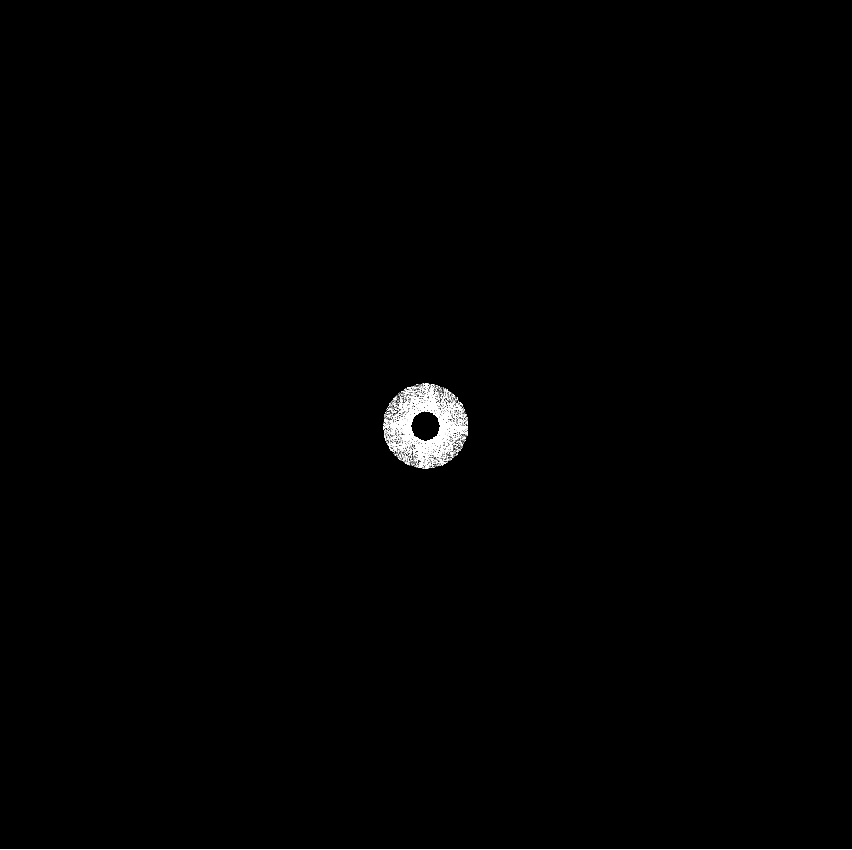
Filtering (滤波)

Filtering (滤波)

Filtering = Getting rid of certain frequency contents (过滤= 摆脱某些频率内容)

• Visualizing Image Frequency Content (可视化图像频率内容)

• Filter Out Low Frequencies Only (Edges) (滤除低频(边缘))

  高通滤波: 只显示高频信息（只显示边界-锐化，将低频信息盖住)

• Filter Out High Frequencies (Blur) (滤除高频(模糊))

  低通滤波: 只显示低频滤波（画面变模糊，将高频信息盖住)

• Filter Out Low and High Frequencies (滤除低频和高频)

Convolution (卷积)

Convolution (卷积)

• Filtering = Convolution (= Averaging) (滤波=卷积 (=平均))

  

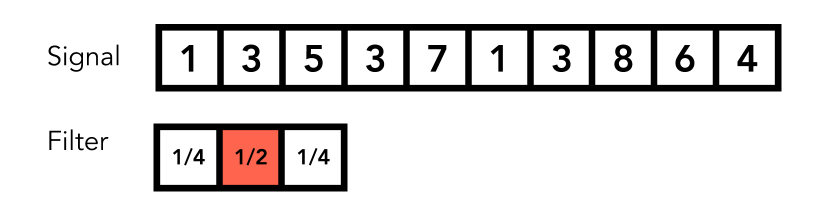
  Point-wise local averaging in a “sliding window”(“滑动窗口”中的逐点局部平均)

  

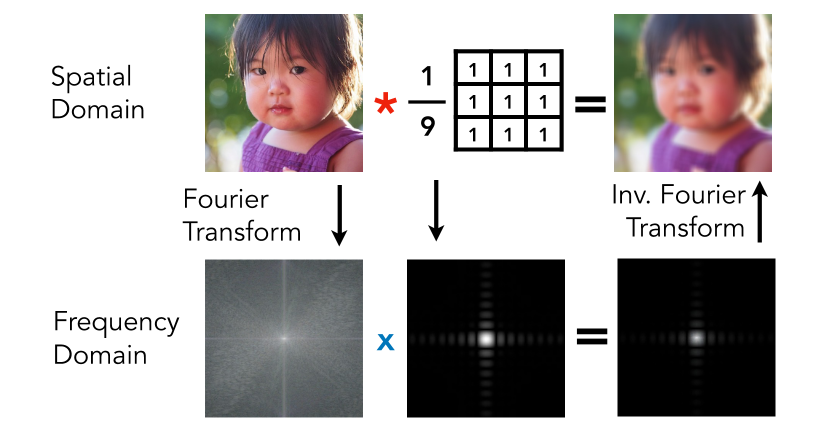
• Convolution Theorem (卷积定理)

  简化的定义: 结果为相邻数的平均值

  Convolution Theorem: Convolution in the spatial domain is equal to multiplication in the frequency domain, and vice versa (卷积定理): 时域的卷积等于频域的乘积

  • Option 1:
    • Filter by convolution in the spatial domain (在空间域中通过卷积滤波)
  • Option 2:
    • Transform to frequency domain (Fourier transform) (变换到频域(傅里叶变换))
    • Multiply by Fourier transform of convolution kernel (乘以卷积核的傅里叶变换)
    • Transform back to spatial domain (inverse Fourier) (变换回空间域(傅里叶反变换))

• Box Filter

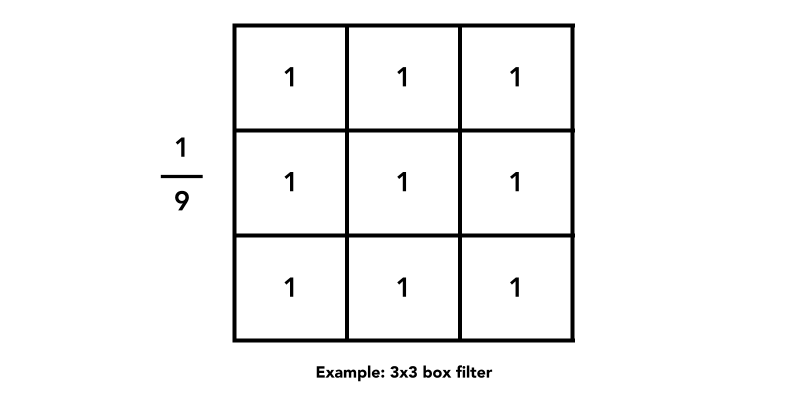
  • Box Function = “Low Pass” Filter
  
  • Wider Filter Kernel = Lower Frequencies
  

来源及解决

INFO

• Sampling = Repeating Frequency Contents (采样=重复频率内容)

  

  From: https://www.researchgate.net/figure/The-evolution-of-sampling-theorem-a-The-time-domain-of-the-band-limited-signal-and-b_fig5_301556095

• Aliasing = Mixed Frequency Contents (混叠=混合频率内容)

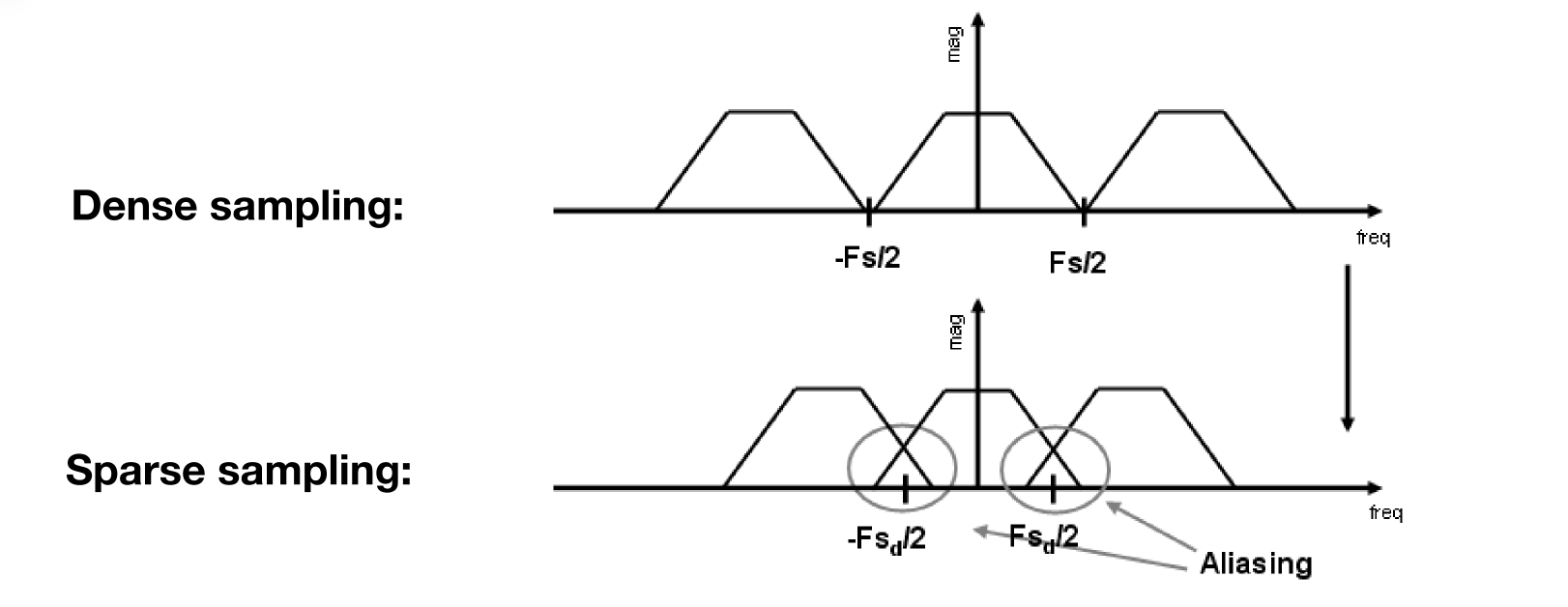
  由于采样稀疏，因此出现频谱混叠从而出现锯齿（走样）如果屏幕中像素非常多，密集的采样就不容易出现走样。

  因此使用分辨率高的显示器，频谱的搬移间隔大，不容易出现频谱混叠。

TIP

• How Can We Reduce Aliasing Error?

  • Option 1: Increase sampling rate (提高采样率)
    • Essentially increasing the distance between replicas in the Fourier domain (本质上是增加了傅立叶域中副本之间的距离)
    • Higher resolution displays, sensors, framebuffers… (更高分辨率的显示器、传感器、帧缓冲区……)
    • But: costly & may need very high resolution (但是:昂贵并且可能需要非常高的分辨率)
  • Option 2: Antialiasing (反走样)
    • Making Fourier contents “narrower” before repeating (在重复之前使傅里叶内容“更窄”)
    • i.e. Filtering out high frequencies before sampling (即: 在采样前滤除高频)

• Regular Sampling (常规取样)

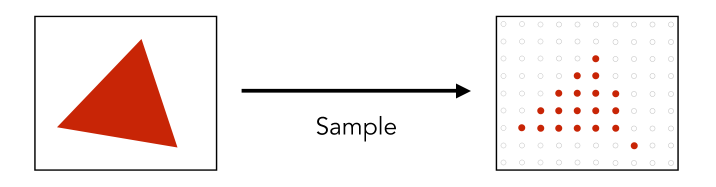
• Antialiased Sampling (反走样采样)

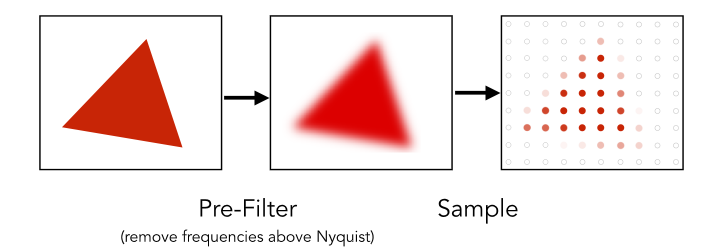
• Antialiasing By Averaging Values in Pixel Area (通过在像素区域的平均值来反走样)

  • Solution:
    • Convolve f(x,y) by a 1-pixel box-blur (对f(x,y)进行1像素的框模糊卷积)
      • Recall: convolving = filtering = averaging (卷积=滤波=平均)
    • Then sample at every pixel’s center (然后再对每个像素的中心取样)

  In rasterizing one triangle, the average value inside a pixel area of f(x,y) = inside(triangle,x,y) is equal to the area of the pixel covered by the triangle. (在光栅化一个三角形时，f(x,y) = inside(triangle,x,y)在像素区域内的平均值等于该三角形所覆盖的像素面积。)

• Antialiasing By Supersampling (MSAA) (通过超采样抗锯齿 (MSAA))

  MSAA: Antialiasing By Supersampling

  Approximate the effect of the 1-pixel box filter by sampling multiple locations within a pixel and averaging their values (通过采样一个像素内的多个位置并取其值的平均值来近似1像素盒滤波器的效果)

  

  ---

  
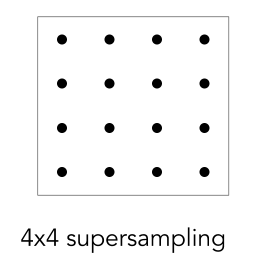
  • Supersampling: Step 1 Take NxN samples in each pixel.
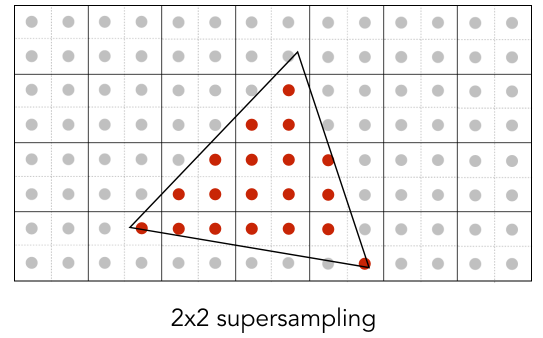
  • Supersampling: Step 2 Average the NxN samples “inside” each pixel.
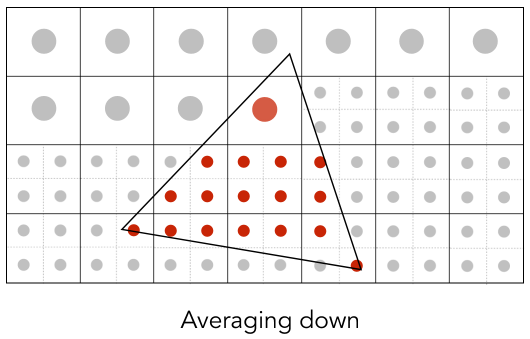
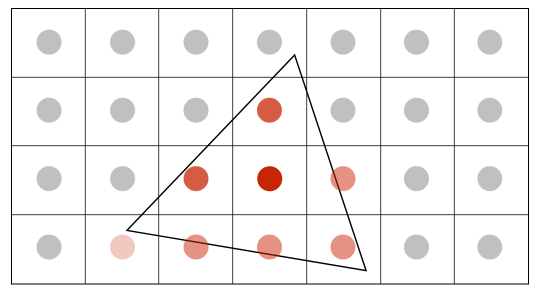
  • Supersampling: Result This is the corresponding signal emitted by the display

结尾

• What’s the cost of MSAA?

  增加了很多的计算量

• 其他的抗锯齿方法

  • FXAA (Fast Approximate AA)
  • TAA (Temporal AA)

• Super resolution / super sampling (Super resolution / super sampling)

  • From low resolution to high resolution (从低分辨率处理成高分辨率)
  • Essentially still “not enough samples” problem (本质上仍然是“样本不足”的问题)
  • DLSS (Deep Learning Super Sampling) (目前可使用DLSS进行超级采样)

Visibility / occlusion (可见性/遮挡)

• Z-buffering (深度缓冲)

Painter’s Algorithm (画家算法)

Inspired by how painters paint Paint from back to front, overwrite in the framebuffer. (灵感来自画家的绘画方式 从后往前涂， 覆盖在帧缓冲区中)

Requires sorting in depth (O(n log n) for n triangles) Can have unresolvable depth order (需要深度排序(O(n log n)对于n个三角形) 会不会有不可解析的深度顺序)

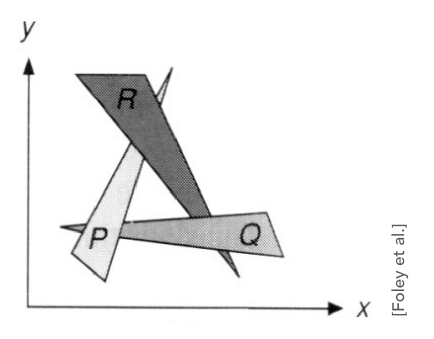
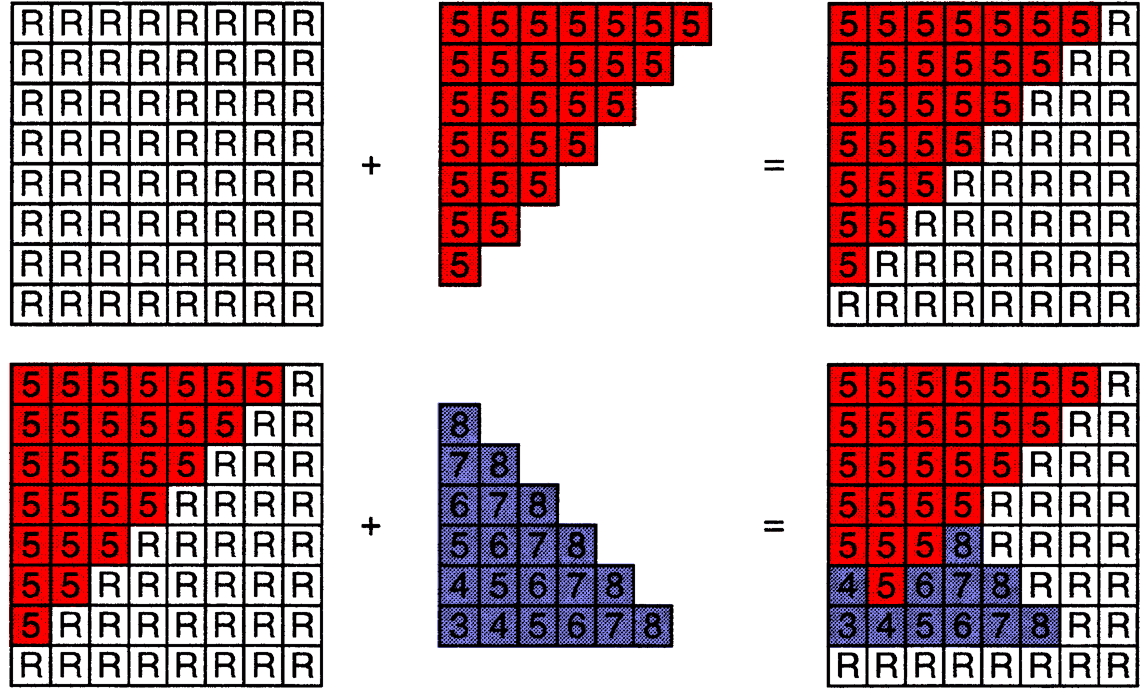
为了解决画家算法的问题, 引入了深度缓冲(Z-Buffer)

Z-Buffer (深度缓冲)

This is the algorithm that eventually won.

• Idea:
  • Store current min. z-value for each sample (pixel) (存储当前最小z值为每一个样本(像素))
  • Needs an additional buffer for depth values (需要一个额外的深度值缓冲区)
    • frame buffer stores color values (帧缓冲区存储颜色值)
    • depth buffer (z-buffer) stores depth (深度缓冲区(z-buffer)存储深度)

MPORTANT: For simplicity we suppose z is always positive (smaller z -> closer, larger z -> further) 永远为正(小->近, 大->远)

示例:

Code:

//默认深度为无限远
for（each triangle T）
{
    for(each sample(x,y,z) in T)//遍历任意一个三角形中的任意一个像素
    {
        if(z<zbuffer[x,y])  //closest sample so far(目前最接近的样本)
        {
            framebuffer[x,y] = rgb;     //update color
            zbuffer[x,y] = z;   //update depth
        }
        else
        {
            ...
        }
    }
}

Z-Buffer Complexity

Complexity

• O(n) for n triangles (assuming constant coverage)
• How is it possible to sort n triangles in linear time

Drawing triangles in different orders?

Most important visibility algorithm

• Implemented in hardware for all GPUs